Alfisol, Item 003: LMMpro, version 2.0
The Langmuir Optimization Program
plus
The Michaelis-Menten Optimization Program
HOME
The Langmuir
Equation
The Michaelis-Menten
Equation
Product Details

Correlation Coefficient (η*2)

The goodness-of-fit of a curve to a given set of data is based on how it compares with the goodness-of-fit of a simple horizontal line at the arithmetic average of the data. The goodness-of-fit of a curve to a set of data points is numerically expressed by the correlation coefficient (η*2).

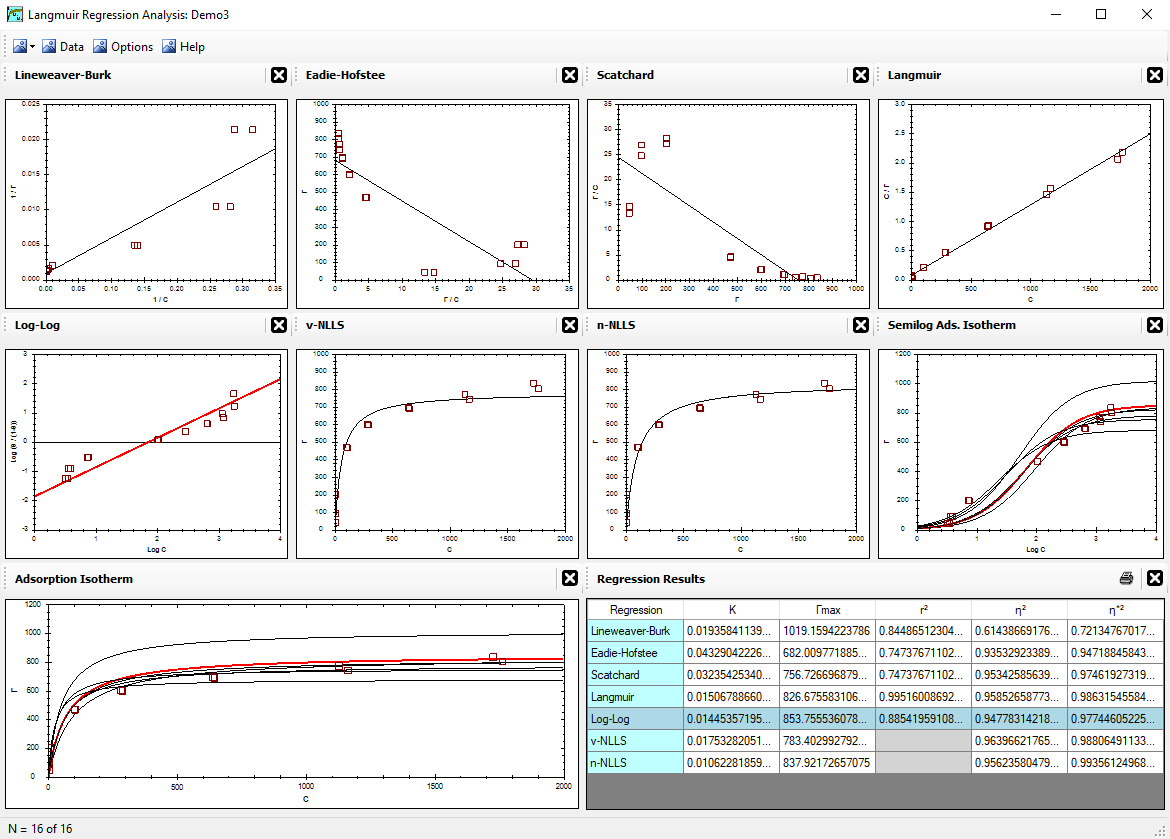
The eta-star-square term is used here for the correlation coefficient value of the untransformed data.

The η*2 assumes that a normal minimum for the error terms corresponds to a better curve fit of the data collected. It is defined as:

η*2 =1 - Σ Qi2
Σ (yi - ya)2
where,
Qi = the normal distance of the datum point
to the nearest point on the predicted curve,

ya = arithmetic mean value of y for all values of x, and
yi = the experimental value measured when x = xi.

A perfect fit by a curve will have η*2 = 1.00, whereas a poor fit by a curve will have a low value. If η*2 = 0, then the predicted curve is no better than a simple average of the data collected. With all regressions, η*2 < 1, always.

This is too difficult to do by hand. Computers are needed to perform these calculations easily and quickly.